Lot of mathematicians have proved Pythagoras theorem in their own ways. If you google it you will indeed found hundred of ways.
Meanwhile I was also sure that maybe one day I could find something new out of this incredible Pythagoras theorem and Recently I got something which I would like to share with you.
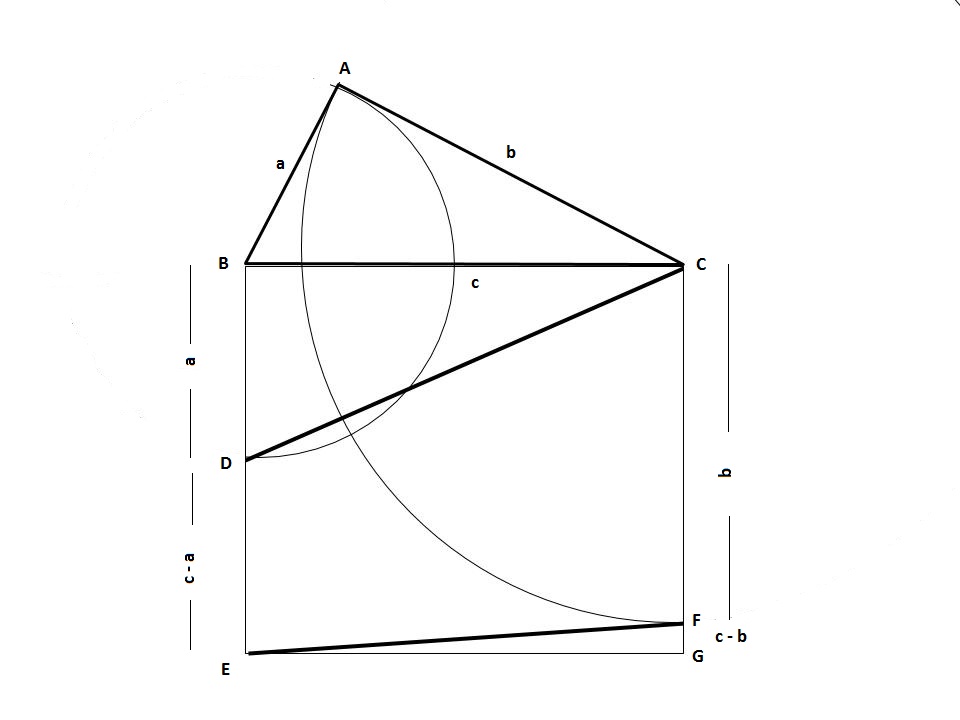
To Prove: Deriving the equation of area of trapezium using Arcs
Proof: There is a triangle ABC with sides a b and c as shown in the figure.
Now,
Area of ∆ BCEG = Area of ∆ BDC +Area of ⌂ DCEF + Area of ∆ EFG
c^2=ac/2+ Area of ⌂ DCEF + (c-b) c/2
(2c^2– ac –c^2+ bc )/2=Area of ⌂ DCEF
(c^2– ac+ bc )/2=Area of ⌂ DCEF
c(c– a+ b)/2=Area of ⌂ DCEF
Area of ⌂ DCEF=BC(DE+CF)/2
Copyrighted©PiyushGoel
contact@piyushgoel.in



